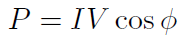There is a long history of modelling electric power transmission systems. The traditional view has been that power cannot be efficiently stored and the partially controllable supply and exogenous demand have to be matched at all times, through the transmission of power generated at generators to substation (transmission system), and eventually, from substations to the individual customers (distribution system). This view is now being challenged by the increasing availability of demand response management, distributed generation, and storage, but electric power transmission is still of paramount importance.
The electric power transmission can be implemented using a variety of means, with the most common one being the overhead power lines. In overhead power lines, pylon are connected by most often multiple high tension lines, each of which is typically made of aluminium wires wrapped around a steel core. Possibly, the may be additional sensors along the line, such as fiber optics for capturing the temperature gradient. One typically utilises the alternating current (AC), whose direction alternates over time. See below for a discussion how this can be modelled.
Compared to overhead power lines, underground and submarine power transmission have much higher investment costs and often higher operational costs. First, underground or under sea, one uses high-voltage (HV) cables with considerable amounts of insulation (often based on pressurised oil or polyethylene). Second, alternating current allows only for very short lines (under 50 km), due to the high capacitance of the cable. While high-voltage direct current (HVDC), where the direction of current is constant over time, requires considerable investment in (and losses at) the power electronics involved in the AC-DC and DC-AC conversion (known as rectifiers and inverters, respectively). While HVDC cables are widely deployed in certain situations already, cf. the deployment of underground power transmission and distribution in Denmark and cables between Germany and Sweden (Baltic Cable), and Norway and the Netherlands (NorNed), it is not clear whether the use of HVDC will spread beyond these situations.
Energy storage is a very active are of research within electrical engineering. Current implementations are based on pumped hydro storage, cf. 10 percent of the peak load in Norway, and pilot projects involving lithium-ion batteries, cf. deployments in New England. The reach of pumped hydro is limited to areas with the appropriate physical geography, while the battery-based systems seem too expensive to operate at scale. Many novel ideas have hence been experimented with, ranging from spinning rotary machinery to moving railway engines uphill, and and it is not clear what shape and form energy storage would take, eventually.
Finally, in demand response management (DRM), one hopes to "emulate" energy storage by incentivising customers to amend their consumption in real time. Although the first related policies have been proposed decades ago, large-scale deployments are still rather limited to, e.g., deferrable loads such as industrial refrigeration. Still, DRM excites many, due to its zero losses, and hence costs bounded from below only by zero.
Models
Although in general, the current and voltage are an arbitrary signal in both alternating and directed current systems, and one could hence use signal processing throughout, one often assumes the harmonic currents to simplify the modelling of real-world power systems. There, voltage, current, and power are sine waves, e.g.
with magnitudes , angular frequency ω, and φ is the phase (offset between current and voltage). (Notice that one can use Fourier transform to approximate any signal by sinusoids). Then, we have a closed-form solution for integral for the average power transmitted, which is equal to the product of the current and the voltage and the cosine of the phase:
Together with the usual relationships of:
- ohmic heating (i.e., losses equal to the product of the resistance and the square of current),
- Kirchhoff's current laws (e.g., sum of current injected is the sum of currents ejected, modulo losses),
one can formulate a variety of mathematical models for the harmonic currents, all of which are non-convex.
A key choice in formulating a mathematical model of harmonic currents is the choice of sine-waves to represent and the choice between polar and rectangular representation thereof. Generally speaking, using the rectangular representation, one can often derive a polynomial optimisation problem, while using the polar representation, one obtains a problem with trigonometric constraints. In some cases, it may also be beneficial to combine both representations, especially when one considers piece-wise linearisations. The key choices studied so far include:
- rectangular power and polar voltage, where power generated at generators and all voltages (except for a reference bus) are employed
- rectangular power and voltage, where power generated at generators and all voltages (except for a reference bus) are employed
- rectangular current and voltage, where currents and voltages are represented
- rectangular current injection.
Although the choice of variables does not affect the results, if exact computation is possible, considering the non-convexity, one often observes a widely varying quality of approximations of a particular type across the choices.
As in much of optimisation and control, one often considers convex relaxations (e.g. semidefinite programming) or mixed-integer convex approximations (e.g. piece-wise linearisations). The most common combinations of variables and approximations include:
- rectangular power and polar voltage can be piece-wise linearised in either an inexact and well-performng or asymptotically exact and rather less well performing fashion
- rectangular power and voltage yields very strong semidefinite-programming relaxations, and convergent hierarchies of semidefinite-programming relaxations
- rectangular current and voltage, which produces weaker convex relaxations, but may be suitable for the use in optimal transmission switching and network expansion planning, where the current may be set to zero without consider a high-degree polynomial
- rectangular current injection, which may again be suitable for the use in optimal transmission switching and network expansion planning, whenever the degree of a polynomial is less of a concern than the dimension of the system.
It should be noted that the convex and piece-wise convex approximations are an active are of research and (m)any rules of thumb above may be invalidated yet. Finally, one sometimes uses the so called "transportation models", where network flows of units of energy are considered.
Contributor:
Dr Jakub Marecek, IBM